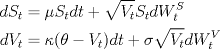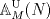 I2 Álgebra Abstracta I, 2S 2009 |
|
|
|
|
|
|
Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Católica > Álgebra Abstracta  |
  Oct 15 2009, 07:42 PM
Publicado:
#1
|
|
 Dios Matemático Supremo  Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:   |
![TEX: \noindent \\<br />\begin{center}MLM2205 - Álgebra Abstracta I\\<br />15 de Octubre de 2009\end{center}<br />\begin{enumerate}<br />\item Demuestre o dé un contraejemplo:<br />\begin{enumerate}<br />\item Si $N\unlhd G$ y $H\leq G$, entonces $H\cap N\unlhd H$.<br />\item $S_3$ tiene tres clases de conjugación.<br />\item Existen grupos simples de orden $200$.<br />\item Sea $G=\left\{A\in M_n(\mathbb{R}):|A|\not= 0\right\}$. Si $G$ actúa sobre $G$ con la acción dada por $A(X)=AX$, existen dos órbitas.<br />\item Existe un anillo conmutativo no unitario con divisores del cero.<br />\item Si $R$ es un anillo de división, entonces $R$ no tiene divisores del cero.<br />\end{enumerate}<br />\item\begin{enumerate}<br />\item Considere la acción del grupo $G=\left\{A=\left[\begin{tabular}{cc}$1$&$0$\\$a$&$b$\end{tabular}\right]:a,b\in\mathbb{Z}_3,|A|\not=0\right\}$ sobre $X=\left\{<\left[\begin{tabular}{c}$1$\\$0$\end{tabular}\right]>,<\left[\begin{tabular}{c}$0$\\$1$\end{tabular}\right]><\left[\begin{tabular}{c}$1$\\$2$\end{tabular}\right]>,<\left[\begin{tabular}{c}$1$\\$1$\end{tabular}\right]>\right\}$ dada por <br />\[A<u>=<Au>.\]<br />Escriba las órbitas, $X_0$ y la fórmula de clase asociada.<br />\item Sea $G$ un grupo de orden 30. Demuestre que $G$ no es simple.<br />\item Determine todos los grupos de orden 1225 que existen.<br />\end{enumerate}<br />\item \begin{enumerate}<br />\item Sea $G$ un grupo, $H,K\in\operatorname{Syl}_p(G)$ y $N\unlhd G$ tal que $H\leq N$.<br />\begin{enumerate}<br />\item Demuestre que existe $x\in N$ tal que $xHx^{-1}=K$.<br />\item Demuestre que $G=N_G(H)\cdot N$.<br />\end{enumerate}<br />\item Sea $R$ un anillo conmutativo y $a\in\mathbb{R}$. Demuestre que el siguiente conjunto es un ideal de $R$<br />\[I_a=\left\{x\in R:\exists y\in R\text{ tal que }x=ya\right\}.\]<br />\end{enumerate}<br />\end{enumerate}<br />](./tex/55d2cff254cee18527badfd700f1a77a.png)
-------------------- |
 |
 
|
![TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]](./tex/e0e88dba678ca3ead120b088f41191b2.png)
|
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 30th April 2025 - 09:59 AM |
