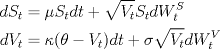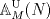 I3 Álgebra Abstracta I, 2S 2009 |
|
|
|
|
|
|
Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Católica > Álgebra Abstracta  |
  Nov 12 2009, 06:56 PM
Publicado:
#1
|
|
 Dios Matemático Supremo  Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:   |
![TEX: \noindent \\<br />\begin{center}MLM2205 - Álgebra Abstracta I\\<br />12 de Noviembre de 2009\end{center}<br />\begin{enumerate}<br />\item Demuestre o dé un contraejemplo:<br />\begin{enumerate}<br />\item $f:\mathbb{Z}\to\mathbb{Z}$ dado por $f(n)=-n$ es un homomorfismo de anillos.<br />\item El ideal $I=\left\{p(x)\in\mathbb{Z}[x]<img src="style_emoticons/default/tongue.gif" style="vertical-align:middle" emoid=":P" border="0" alt="tongue.gif" />(0)\in 2\mathbb{Z}\right\}$ es maximal de $\mathbb{Z}[x]$.<br />\item El cuerpo de cuocientes de $\mathbb{Z}[x]$ es $\mathbb{Q}[x]$. <br />\item En un dominio $D$, si $x$ es irreducible e $y$ divide a $x$, entonces $y$ es unidad o $x$ es asociado a $y$.<br />\item En $\mathbb{Z}_3[x]/<x^2+x+1>$, el elemento $x+<x^2+x+1>$ es una unidad.<br />\item $\mathbb{Z}_3[x]/<x^2+x+1>$ no es un cuerpo.<br />\end{enumerate}<br />\item\begin{enumerate}<br />\item Determine todos los homomorfismos de anillos $\phi:\mathbb{Q}\to\mathbb{Q}$.<br />\item Sea $R$ un anillo conmutativo, $I$ ideal de $R$ y $X$ un ideal de $R/I$. Demuestre que existe $J$ ideal de $R$ tal que $I\subseteq J$ y $J/I=X$.<br />\item Sea $I=\left\{\frac{a}{b}\in\mathbb{Q}<img src="style_emoticons/default/sad.gif" style="vertical-align:middle" emoid=":(" border="0" alt="sad.gif" />a,b)=1\land p\nmid b\land p|a\right\}$ un ideal del anillo\\ $A=\left\{\frac{a}{b}\in\mathbb{Q}<img src="style_emoticons/default/sad.gif" style="vertical-align:middle" emoid=":(" border="0" alt="sad.gif" />a,b)=1\land p \nmid b\right\}.$ Demuestre que $A/I\cong \mathbb{Z}_p$.<br />\end{enumerate}<br />\item Sean $R$ y $S$ anillos conmutativos, unitarios y $\phi:R\to S$ un epimorfismo.<br />\begin{enumerate}<br />\item Si $J$ es un ideal primo de $S$, demuestre que $\phi^{-1}(J)$ es un ideal primo de $R$ que contiene a $\ker (\phi)$.<br />\item Si $I$ es un ideal primo de $R$ que contiene a $\ker (\phi)$, demuestre que $\phi(I)$ es un ideal primo de $S$.<br />\item Use lo anterior para probar que el ideal de la pregunta $1)b)$ es primo.<br />\end{enumerate}<br />\end{enumerate}<br />](./tex/744bed79f889793dc4e3d5983109828a.png) -------------------- |
 |
 
|
![TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]](./tex/e0e88dba678ca3ead120b088f41191b2.png)
|
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 30th April 2025 - 09:54 AM |
